Measuring Risk: Utility Functions by Daniel Bernoulli

Background on these posts 

Apart from reading books I think we must read Journal Papers. Now there is a problem in doing so - it's complicated, right? Yes they are and I feel they are made to be so deliberately as the end audience is not the common man but rather a specialist. I have read quite a few over the years, some I understood pretty well and some very minimally given my limited mathematical abilities. But in going through these papers I always could derive the essential takeaway - the crux. Also one problem I saw was application. I don't see how all these papers could be applied be it in actual trading or in developing some heuristic for our investing endeavour. Therefore I thought over time I will start doing the following 3 things:

• Crux: Summarise key points in some 'good' papers in plain english
• Simplify: Remove math formulas from the papers as much as possible
• Actionable: Share my ideas on application of the concepts in that paper

Sometimes will throw in some trivia for fun sake. Now let us move on.

Paper: Exposition of a new theory on the measurement of risk

Author: Daniel Bernoulli

Published: 1738 (English translation in 1954)

This is the first paper in the seminal book on the Kelly Criterion. The paper can be found here, some of the sections have been underlined by me. More about Bernoulli here.

Main takeaway

In plain English - The usefulness of additional wealth is dependent on the current wealth as well as the circumstance of a person.

Summary for the layman

If there is a game where a person is given a choice between two situations which modifies his current wealth, which option should the person choose? Earlier this problem was solved by simply calculating expected payoffs. For example if there are two choices, option one which yields 20% increase in wealth with a chance of 30% and option two yields a 10% increase in wealth with a chance of 70%. Which option should you choose? The expected payoff was simply calculated by (20% * 30% = 6% and 10% * 70% = 7%). Thus we should play this game with the second option to increase wealth.

Risk in a gamble can be judged by the financial position in which a person is in currently. Usually the term 'gamble'/'game' is used to mean an investment in such papers. Bernoulli says all men cannot use the same rule to evaluate the gamble.

The value of a good should not be based on its price, it should rather be based on its usefulness to the individual. Thus Bernoulli means that the term 'utility' is a very personal one, meaning not just depending on the financial condition of the person does the utility (i.e. the usefulness of the good) depend but also on his circumstances. For instance the value in a gamble of 1,000,000 rupees could be life changing for a beggar but not a millionaire. The utility for the beggar is immense but not so in the millionaire's case. But what if the millionaire is caught up in a legal case (his circumstance) and he needs to furnish an additional million to get a bail. In this case even the utility of that million for the millionaire is substantial.

So the game of the payoff in the example given above should take utility values rather than the profit expectation from the utilities of each outcome of the game. Bernoulli says that risk cannot be estimated without knowing utilities.

Increase in wealth, no matter how insignificant will result in an increase in utility which is inversely proportional to the current wealth. This statement has important implications when we make bets in investing especially related to position sizing.

A philosophical statement which Bernoulli makes is 'The man who is emotionally less affected by a gain will support a loss with greater patience.'

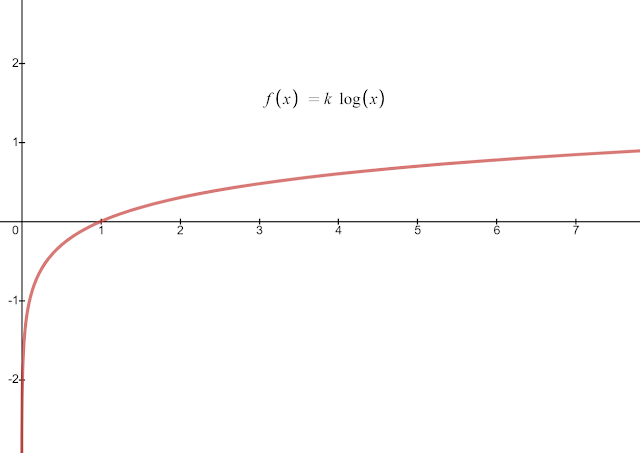
Without delving into the maths used in the paper Bernoulli demonstrates that the utility function is logarithmic in nature (just like the chart at the start of this post). The X axis is wealth and the Y axis is the utility and the red line is the utility function (note that at x = 0 y is at infinity i.e. asymptote). The derivation is given in the paper which I have attached, the math is high school level only.

Finally Bernoulli states that any gain from playing a game must be added to the existing wealth and then raised to the power of the probability of its occurrence. All the possible outcomes should be calculated this way (add to previous wealth and raise to the probability of that occurrence) and multiplied with each other. This product then should be raised to the power of inverse of all  sums of possible of outcomes. The initial wealth should be subtracted to finally arrive at the risk associated with playing that game*.

Got confused? Yes. Ignore the last paragraph. Just read it as - some math is done and risk is calculated which takes into consideration usefulness of each outcome in the game for the individual.

If we are able to calculate utilities of such chance situations we have a good tool at hand in making decisions. Bernoulli gives a couple of examples about whether it makes sense to buy an insurance policy while shipping goods or not. The key to note is that the decision is dependent on the existing wealth of the person like explained previously.

In the second part of the paper he introduces us to the St Petersburg problem. I will skip this as it is only another case of the utility function. But do check out the wikipedia link.

*Note: There is some criticism in the calculation done where we expect a 'fee' to be deducted. But that is the nuts and bolts and we are focusing more on the idea rather than the calculation. It is believed that the translator made some changes in the diagram and interpretation, so I will give Mr Bernoulli the benefit of the doubt.

Application

What could be the application you might be thinking other than being proud that you now understand some academic paper published in the 1700s. The application to investing is in how bet sizing is done. Directly we as long term stock pickers do not have a method of application as such.

The usefulness will kick in when this logarithm function mentioned here is used for bet sizing in the Kelly method. It will help us take decisions on what percentage of our existing wealth we will bet on a given investment (provided that we know certain estimates like expected gain and advantage in the game). By knowing this bet size we will overall try to optimise the total wealth we have. Mathematically it is doable but some of us do this intuitively too - when we have high confidence ('edge' in the game, measured by probability) and expect a high profit ('payoff' in the game, measured by win size to loss size in the game) we bet the 'house' in that investment opportunity (very high allocation in a high probability/confidence scenario with very large potential profits).

In conclusion we ought to remember that utility or the usefulness of additional wealth will depend on current wealth and circumstances. This usefulness will follow the logarithmic curve.

In a continuing post I will share actual methods to maximize geometric returns for index investing for salaried professionals. It is a math experiment which I have been doing. But that is for another day.